若最高处(
v
=
0
{\displaystyle v=0}
θ
{\displaystyle \theta }
θ
≤
5
∘
{\displaystyle \theta \leq 5^{\circ }}
则可使用下列公式算出它的振动周期 。
T
=
2
π
L
g
{\displaystyle T=2\pi {\sqrt {\frac {L}{g}}}}
L
{\displaystyle L}
g
{\displaystyle g}
一摆长为
1
{\displaystyle 1}
简谐运动 ,周期
T
≈
2.0
s
{\displaystyle T\approx 2.0s}
秒摆 。
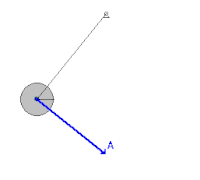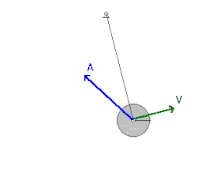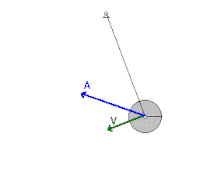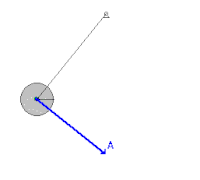
一单摆摆锤正在摆荡最高处(此时
v
=
0
{\displaystyle v=0}
θ
{\displaystyle \theta }
L
{\displaystyle L}
x
{\displaystyle x}
此物体受下列力的影响(下列说明错误,绳子的张力是因为摆锤重力引起,任何一瞬间摆锤法向(径向)合力为零,但切线加速度为
−
g
sin
θ
{\displaystyle -g\sin \theta }
绳子之拉力大小
F
{\displaystyle F}
重力大小
F
g
=
m
g
{\displaystyle F_{g}=mg}
绳子的拉力
F
{\displaystyle F}
F
cos
θ
=
m
g
{\displaystyle F\cos \theta =mg}
F
sin
θ
=
k
x
{\displaystyle F\sin \theta =kx}
∵
lim
θ
→
0
cos
θ
=
1
{\displaystyle \because {\underset {\theta \to 0}{\mathop {\lim } }}\,\cos \theta =1}
∴
F
≈
m
G
g
{\displaystyle \therefore F\approx m_{G}g}
F
sin
θ
=
m
G
g
(
x
L
)
=
k
x
{\displaystyle F\sin {\theta }=m_{G}g\left({\frac {x}{L}}\right)=kx}
k
=
m
G
g
L
{\displaystyle k={\frac {m_{G}g}{L}}}
代入
T
=
2
π
m
I
k
{\displaystyle T=2\pi {\sqrt {\frac {m_{I}}{k}}}}
得到
T
=
2
π
m
I
L
m
G
g
{\displaystyle T=2\pi {\sqrt {\frac {m_{I}L}{m_{G}g}}}}
根据广义相对论 可知,
m
I
=
m
G
{\displaystyle m_{I}=m_{G}\,}
故
T
=
2
π
L
g
{\displaystyle T=2\pi {\sqrt {\frac {L}{g}}}}
sin θ 取为θ的误差。 “
单摆 ”重定向至此。
取
L
{\displaystyle L}
θ
{\displaystyle \theta }
θ
0
{\displaystyle \theta _{0}}
θ
{\displaystyle \theta }
m
{\displaystyle m}
θ
¨
{\displaystyle {\ddot {\theta }}}
α
=
d
2
θ
d
t
2
{\displaystyle \alpha ={\frac {{\rm {d}}^{2}\theta }{{\rm {d}}t^{2}}}}
忽略空气阻力以及绳的弹性、重量的影响:
锤速率最高是在
θ
=
0
{\displaystyle \theta =0}
运动方程为:
m
L
θ
¨
=
−
m
g
sin
θ
{\displaystyle mL{\ddot {\theta }}=-m{\rm {g}}\sin \theta }
注意到不论θ 的值为何,运动周期和锤的质量无关。
当
θ
{\displaystyle \theta }
sin
θ
≈
θ
{\displaystyle \sin \theta \approx \theta }
简谐运动 ,周期
T
=
2
π
L
g
{\displaystyle T=2\pi {\sqrt {\frac {L}{g}}}}
准确的运动周期不可以用基础函数求得。考虑微分方程:
d
t
d
θ
=
1
2
L
g
1
cos
θ
−
cos
θ
0
{\displaystyle {{\rm {d}}t \over {\rm {d}}\theta }={1 \over {\sqrt {2}}}{\sqrt {L \over {\rm {g}}}}{1 \over {\sqrt {\cos \theta -\cos \theta _{0}}}}}
T
=
θ
0
→
0
→
−
θ
0
→
0
→
θ
0
=
4
(
θ
0
→
0
)
{\displaystyle T=\theta _{0}\rightarrow 0\rightarrow -\theta _{0}\rightarrow 0\rightarrow \theta _{0}=4\left(\theta _{0}\rightarrow 0\right)}
T
=
4
1
2
L
g
∫
0
θ
0
1
cos
θ
−
cos
θ
0
d
θ
{\displaystyle T=4{1 \over {\sqrt {2}}}{\sqrt {L \over {\rm {g}}}}\int _{0}^{\theta _{0}}{1 \over {\sqrt {\cos \theta -\cos \theta _{0}}}}\,{\rm {d}}\theta }
将上式重写成第一类椭圆函数 的形式:
T
=
4
L
g
F
(
sin
θ
0
2
,
π
2
)
{\displaystyle T=4{\sqrt {L \over {\rm {g}}}}F\left({\sin {\theta _{0} \over 2}},{\pi \over 2}\right)}
其中
F
(
k
,
ϕ
)
=
∫
0
ϕ
1
1
−
k
2
sin
2
θ
d
θ
.
{\displaystyle F(k,\phi )=\int _{0}^{\phi }{1 \over {\sqrt {1-k^{2}\sin ^{2}{\theta }}}}\,{\rm {d}}\theta .}
周期可以用级数表示成:
T
=
2
π
L
g
[
1
+
(
1
2
)
2
sin
2
θ
0
2
+
(
1
⋅
3
2
⋅
4
)
2
sin
4
θ
0
2
+
(
1
⋅
3
⋅
5
2
⋅
4
⋅
6
)
2
sin
6
θ
0
2
+
⋯
]
{\displaystyle T=2\pi {\sqrt {L \over {\mathrm {g} }}}\left[1+\left({\frac {1}{2}}\right)^{2}\sin ^{2}{\frac {\theta _{0}}{2}}+\left({\frac {1\cdot 3}{2\cdot 4}}\right)^{2}\sin ^{4}{\frac {\theta _{0}}{2}}+\left({\frac {1\cdot 3\cdot 5}{2\cdot 4\cdot 6}}\right)^{2}\sin ^{6}{\frac {\theta _{0}}{2}}+\cdots \right]}
=
2
π
L
g
(
1
+
1
16
θ
0
2
+
11
3072
θ
0
4
+
⋯
)
=
2
π
L
g
[
∑
n
=
0
∞
(
(
2
n
)
!
2
2
n
(
n
!
)
2
)
2
sin
2
n
(
θ
0
2
)
]
{\displaystyle =2\pi {\sqrt {\frac {L}{g}}}\left(1+{\frac {1}{16}}\theta _{0}^{2}+{\frac {11}{3072}}\theta _{0}^{4}+\cdots \right)=2\pi {\sqrt {\frac {L}{g}}}\left[\sum _{n=0}^{\infty }\left({\frac {\left(2n\right)!}{2^{2n}\left(n!\right)^{2}}}\right)^{2}\sin ^{2n}\left({\frac {\theta _{0}}{2}}\right)\right]}
冲击摆是来用计算子弹速度的实验室仪器。它的原理为:物件碰撞前后动量守恒 ,摆运动时能量守恒 。
冲击摆和普通摆相似,特别之处它的锤会和射入子弹产生完全非弹性碰撞,即碰撞后两者会合为一。
将子弹射向停止的锤,使锤和子弹合在一起摆动。设锤质量为
m
p
{\displaystyle m_{p}\,}
m
b
{\displaystyle m_{b}\,}
v ,锤和子弹碰撞后的速度为u 。
以下是子弹速度的计算方法:
由动量守恒定律 ,
m
b
×
v
+
m
p
×
0
=
(
m
b
+
m
p
)
×
u
{\displaystyle m_{b}\times v+m_{p}\times 0=(m_{b}+m_{p})\times u}
由能量守恒定律 ,
1
2
(
m
b
+
m
p
)
u
2
=
(
m
b
+
m
p
)
g
h
{\displaystyle {\frac {1}{2}}(m_{b}+m_{p})u^{2}=(m_{b}+m_{p})gh}
解得
v
=
(
m
b
+
m
p
)
2
g
h
m
b
{\displaystyle v={\frac {(m_{b}+m_{p}){\sqrt {2gh}}}{m_{b}}}}
和台车和倒单摆组成的系统 倒单摆有许多不同的架构,常见的有二种。
最简单的是无质量的直杆一端接在固定的枢纽上,另一端连结重量,此架构类似一般单摆,但因为重量在枢纽点上方,直杆在重量下方,需支持重物不落下,因此会将单摆的线改为有刚性的直杆。
另外一种是将倒单摆放在可以一维水平运动的台车上,透过台车的水平运动来控制摆的位置。
倒单摆在摆直立朝上时可以平衡,不过是不稳定平衡,需要透过控制系统才能维持平衡。
圆锥 面。这是圆周运动 。
编辑
“
复摆 ”重定向至此。
当质量不集中或不规则的物体以转轴吊起摆动时,此摆称作复摆(物理摆)。由于有质量分布的缘故,周期跟刚性物体重心对转轴的转动惯量(I)有关。根据平行轴定理及可以求出小角度复摆周期为
T
=
2
π
I
m
g
d
{\displaystyle T=2\pi {\sqrt {\frac {I}{mgd}}}}
编辑
双摆系统的一例 双摆系统是混沌的。
和双摆一样,磁性摆系统是混沌的。