旋量
此条目需要精通或熟悉相关主题的编者参与及协助编辑。 (2017年12月10日) |
在数学几何学与物理中,旋量(spinor)是复向量空间中的元素。旋量乃自旋群的表象,类似于欧几里得空间中的向量以及更广义的张量,当欧几里得空间进行无限小旋转时,旋量做相应的线性转换。当如此一系列这样的小旋转组合成一定量的旋转时,这些旋量转换的次序会造成不同的组合旋转结果,与向量或张量的情形不同。当空间从0°开始,旋转了完整的一圈(360°),旋量发生了正负号变号(见图),这个特征即是旋量最大的特点。在一给定维度下,需要旋量才能完整地描述旋转,如此引入了额外数量的维度。
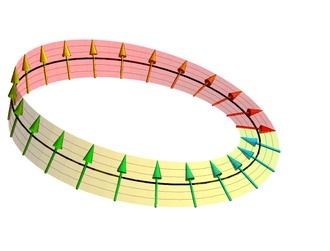
在闵考斯基空间的情形,也可以定义出相似的旋量,其中狭义相对论的劳仑兹转换扮演旋转的角色。旋量最先是由埃利·嘉当于1913年引入几何学。[1][2]在1920年代,物理学家发现若要描述电子及其他次原子粒子的内禀角动量或自旋,旋量为不可或缺的角色。旋量群为所有旋转相关的旋量所构成的群,其二重覆叠了旋转群,因为每个完整旋转结果皆有两种不同但等效的旋转方式。
概论
编辑一种特定的旋量是旋转群(李群SO(n,R))的投影表示中的元素,或更广义地说,是SO(p,q,R)群的投影表示中的元素。
旋量常被描述成“向量的平方根”,因为向量表示会出现在两个相同旋量表示的张量积。
旋量中最典型的是狄拉克旋量。
数学性质
编辑当前有两种架构可建构出旋量。
一者是表示论架构。正交群的李代数中,有一些群表示无法以寻常的张量来建构,这些群表示称之为旋量群表示,组成成分即旋量。在此观点下,旋量属于旋转群的二重复叠的表示SO(n, R);更广义的情形,其为度规记号为(p, q)之空间中,广义特殊正交群的二重复叠SO+(p, q, R)。这些二重复叠为称作旋量群Spin(n)或Spin(p, q)的李群。
二者是几何架构。人们可以直接建构旋量,并检视相关李群操作下旋量的行为。此方法的优点是直观,但对旋量的复杂性质则难以处理,例子包括菲尔兹恒等式。
克里福代数
编辑自旋群
编辑物理学中的名词
编辑表示论中的旋量
编辑历史
编辑埃利·嘉当于1913年提出旋量的最广义数学形式。[3]“旋量”一词则是首先出现在保罗·埃伦费斯特的量子物理论文中。[4]
1927年,沃尔夫冈·包立将旋量应用至数学物理,当时他引入了自旋矩阵。[5]隔年,保罗·狄拉克发现了相对论性的电子自旋理论,其中展示了旋量与劳仑兹群的关连。[6]于1930年代,狄拉克、皮亚特·海恩以及玻尔研究所的其他研究者建立了Tangloids之类的玩具,作为旋量的教学以及旋量微积分的模型。
建构
编辑克莱布希-高登系数
编辑低维度总结
编辑相关条目
编辑参考文献
编辑- ^ Cartan 1913.
- ^ Quote from Elie Cartan: The Theory of Spinors, Hermann, Paris, 1966, first sentence of the Introduction section of the beginning of the book (before the page numbers start): "Spinors were first used under that name, by physicists, in the field of Quantum Mechanics. In their most general form, spinors were discovered in 1913 by the author of this work, in his investigations on the linear representations of simple groups*; they provide a linear representation of the group of rotations in a space with any number of dimensions, each spinor having components where or ." The star (*) refers to Cartan 1913.
- ^ Cartan 1913
- ^ Tomonaga 1998,第129页
- ^ Pauli 1927.
- ^ Dirac 1928.
书目
编辑- Brauer, Richard; Weyl, Hermann, Spinors in n dimensions, American Journal of Mathematics (The Johns Hopkins University Press), 1935, 57 (2): 425–449, JSTOR 2371218, doi:10.2307/2371218.
- Cartan, Élie, Les groupes projectifs qui ne laissent invariante aucune multiplicité plane (PDF), Bul. Soc. Math. France, 1913, 41: 53–96 [2015-05-29], (原始内容存档 (PDF)于2017-06-29).
- Cartan, Élie, The theory of spinors, Paris, Hermann (reprinted 1981, Dover Publications), 1966, ISBN 978-0-486-64070-9
- Chevalley, Claude, The algebraic theory of spinors and Clifford algebras, Columbia University Press (reprinted 1996, Springer), 1954, ISBN 978-3-540-57063-9.
- Dirac, Paul M., The quantum theory of the electron, Proceedings of the Royal Society of London, 1928, A117: 610–624, JSTOR 94981.
- Fulton, William; Harris, Joe, Representation theory. A first course, Graduate Texts in Mathematics, Readings in Mathematics 129, New York: Springer-Verlag, 1991, ISBN 0-387-97495-4, MR 1153249.
- Gilkey, Peter B., Invariance Theory, the Heat Equation, and the Atiyah–Singer Index Theorem, Publish or Perish, 1984 [2015-05-29], ISBN 0-914098-20-9, (原始内容存档于2020-07-06).
- Harvey, F. Reese, Spinors and Calibrations, Academic Press, 1990, ISBN 978-0-12-329650-4.
- Hazewinkel, Michiel (编), Spinor, 数学百科全书, Springer, 2001, ISBN 978-1-55608-010-4
- Hitchin, Nigel J., Harmonic spinors, Advances in Mathematics, 1974, 14: 1–55, MR 0358873, doi:10.1016/0001-8708(74)90021-8.
- Lawson, H. Blaine; Michelsohn, Marie-Louise, Spin Geometry, Princeton University Press, 1989, ISBN 0-691-08542-0.
- Pauli, Wolfgang, Zur Quantenmechanik des magnetischen Elektrons, Zeitschrift für Physik, 1927, 43 (9–10): 601–632, Bibcode:1927ZPhy...43..601P, doi:10.1007/BF01397326.
- Penrose, Roger; Rindler, W., Spinors and Space–Time: Volume 2, Spinor and Twistor Methods in Space–Time Geometry, Cambridge University Press, 1988, ISBN 0-521-34786-6.
- Tomonaga, Sin-Itiro, Lecture 7: The Quantity Which Is Neither Vector nor Tensor, The story of spin, University of Chicago Press: 129, 1998, ISBN 0-226-80794-0