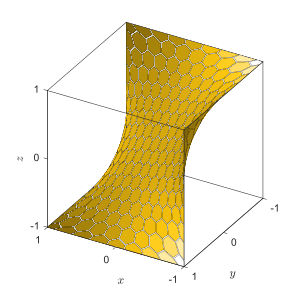
猴鞍面
數學中,猴鞍面(monkey saddle)是方程

猴鞍面屬於鞍面,名稱來源於這樣的觀察:給猴子準備的鞍需要兩個凹陷來放腿,一個凹陷來放尾巴。猴鞍面的原點對應在原點的退化臨界點。猴鞍面有一個孤立的臍點,原點處的高斯曲率為零,而在所有其他點的曲率都是嚴格負的。 可通過複數將直角坐標與圓柱坐標聯繫起來:
將圓柱方程中的3替換為任意整數,就可以得到具有k凹陷的鞍面。 [1]
猴鞍面的另一個方向是由定義的Smelt花瓣,因此猴鞍面的z軸對應Smelt花瓣中的方向。[2][3]
馬鞍面
編輯馬鞍面(horse saddle)指 在x-y平面的每個方向上都有一個鞍點、局部最小值或最大值的普通鞍面。相比之下,猴鞍面在每個方向上都有靜止的拐點。
參考文獻
編輯- ^ Peckham, S.D. (2011) Monkey, starfish and octopus saddles, Proceedings of Geomorphometry 2011, Redlands, CA, pp. 31-34, https://www.researchgate.net/publication/256808897_Monkey_Starfish_and_Octopus_Saddles
- ^ J., Rimrott, F. P. Introductory Attitude Dynamics. New York, NY: Springer New York. 1989: 26. ISBN 9781461235026. OCLC 852789976.
- ^ Chesser, H.; Rimrott, F.P.J. Rasmussen, H. , 編. Magnus Triangle and Smelt Petal. CANCAM '85: Proceedings, Tenth Canadian Congress of Applied Mechanics, June 2-7, 1985, the University of Western Ontario, London, Ontario, Canada. 1985.



![{\displaystyle z=x^{3}-3xy^{2}=\operatorname {Re} [(x+iy)^{3}]=\operatorname {Re} [r^{3}e^{3i\varphi }]=r^{3}\cos(3\varphi ).}](https://wikimedia.org/api/rest_v1/media/math/render/svg/1f220476d5da875b85983730239cae29ae72bc9e)



