決定系數

決定系數，或稱判定系數（英語：Coefficient of determination，記為R ²），在統計學中用於度量應變量的變異中可由自變量解釋部分所佔的比例，以此來判斷迴歸模型的解釋力。

決定系數$R^{2}=1-{\frac {\color {blue}{SS_{\text{res}}}}{\color {red}{SS_{\text{tot}}}}}$示意圖 線性迴歸（右側）的效果比起平均值（左側）越好，決定系數的值就越接近於1。 藍色正方形表示線性迴歸的殘差的平方， 紅色正方形數據表示對於平均值的殘差的平方。

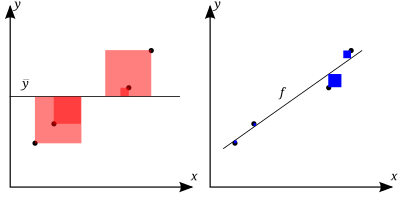
對於簡單線性迴歸而言，決定系數為樣本相關係數的平方。當加入其他迴歸自變量後，決定系數相應地變為多重相關係數的平方。

假設一數據集包括y₁,...,y_n共n個觀察值，相對應的模型預測值分別為f₁,...,f_n。定義殘差e_i = y_i − f_i，平均觀察值為

${\bar {y}}={\frac {1}{n}}\sum _{i=1}^{n}y_{i}.$

於是可以得到總平方和

$SS_{\text{tot}}=\sum _{i}(y_{i}-{\bar {y}})^{2},$

殘差平方和

$SS_{\text{res}}=\sum _{i}(y_{i}-f_{i})^{2}=\sum _{i}e_{i}^{2}\,$

由此，決定系數可定義為

$R^{2}\equiv 1-{SS_{\rm {res}} \over SS_{\rm {tot}}}.\,$