配邊

在數學中，配邊（英文：cobordism 來自法文的 bord）是緊流形的等價關係。它使用邊界的拓撲概念。若兩個流形M和N的不交並是另一個流形W的邊界，那麼M和N這兩個流形是配邊的。此外M和N的配邊是W：

$\partial W=M\sqcup N$ .

配邊縮寫為 $(W;M,N)$ 。M的配邊類（cobordism class）是與M配邊的所有流形的集合。 ^[1]

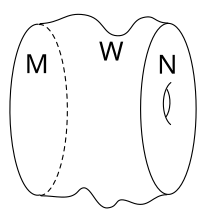
例子

最簡單的例子是區間 I =[0,1]。這是 {0}和{1}這兩個0-維流形的1-維配邊。

Pair of pants的配邊

如果M 是圓，N是兩個圓, 那麼M 和 N 的不交並是pair of pants（W）的邊界。所以pair of pants是M和N的配邊。

3維配邊

W=\mathbb {S} ^{1}\times \mathbb {D} ^{2}-\mathbb {D} ^{3}

；

M=\mathbb {S} ^{2}

是0-維流形；

N=\mathbb {S} ^{1}\times \mathbb {S} ^{1}

是2-環面（見割補理論）

參見

腳註

^ 若M和N是 $n$ 維的，則W是 $(n+1)$ 維的，而且這是 $(n+1)$ 維的配邊。

參考文獻

John Frank Adams, Stable homotopy and generalised homology, Univ. Chicago Press (1974).
Anosov, Dmitri; bordism
米高·阿蒂亞, Bordism and cobordism Proc. Camb. Phil. Soc. 57, pp. 200–208 (1961).
Dieudonne, Jean Alexandre. A history of algebraic and differential topology.
Kosinski, Antoni A. Differential Manifolds. Dover Publications. October 19, 2007.
Madsen, Ib. The classifying spaces for surgery and cobordism of manifolds. 普林斯頓
約翰·米爾諾，A survey of cobordism theory.
謝爾蓋·彼得羅維奇·諾維科夫, Methods of algebraic topology from the point of view of cobordism theory, Izv. Akad. Nauk SSSR Ser. Mat. 31 (1967), 855–951.
列夫·龐特里亞金, Smooth manifolds and their applications in homotopy theory American Mathematical Society Translations, Ser. 2, Vol. 11, pp. 1–114 (1959).
丹尼爾·奎倫, On the formal group laws of unoriented and complex cobordism theory Bull. Amer. Math. Soc., 75 (1969) pp. 1293–1298.
Douglas Ravenel, Complex cobordism and stable homotopy groups of spheres, Acad. Press (1986).
Yuli Rudyak Cobordism.
Yuli B. Rudyak, On Thom spectra, orientability, and (co)bordism, Springer (2008).
Robert E. Stong, Notes on cobordism theory, Princeton Univ. Press (1968).
Taimanov, Iskander. Topological library. Part 1: cobordisms
勒內·托姆, Quelques propriétés globales des variétés différentiables, Commentarii Mathematici Helvetici 28, 17-86 (1954).
Wall, C. T. C. Determination of cobordism ring. Annals of Mathematics（數學年刊）
Bordism on the Manifold Atlas.
B-Bordism Archive.is的存檔，存檔日期2012-05-29 on the Manifold Atlas.

[1] 若M和N是 $n$ 維的，則W是 $(n+1)$ 維的，而且這是 $(n+1)$ 維的配邊。

[1]