莫尔圆

莫尔圆（Mohr's circle）得名自德国土木工程师克里斯汀·奥图·莫尔，是一种用二维方式表示柯西应力张量转换关系的图。

图1：三维应力下的莫尔圆

先针对假设为连续的物体进行应力分析，之后特定一点的柯西应力张量分量会和坐标系有关。莫尔圆是用图形的方法去确认一个旋转坐标系上的应力分量，也就是在同一点上，但是作用在不同方向平面上的分量。

圆上每一个点的横坐标$\sigma _{\mathrm {n} }$及纵坐标$\tau _{\mathrm {n} }$都是在这个旋转坐标系统上某一个方向的正应力及剪应力。换句话说，莫尔圆表示了在所有方向平面上应力状态的轨迹，而X轴和Y轴为应力元素的主轴。

卡尔·卡尔曼是第一个想到用图形来表示应力的人，他是在分析水平梁承受弯曲时的纵向应力及垂直应力时所想到的。莫尔的贡献不止是用莫尔圆表示二维及三维的应力，他也根据莫尔圆发展了结构失效判定的准则。

其他表示应力状态的方式有拉梅应力椭球及柯西应力二次曲线（Cauchy's stress quadric）。

莫尔圆可以扩展到对称的 2x2 张量，包括应变及转动惯量张量。

应力及莫尔圆

图2：在有受力可变形物体（假设为连续体）中的应力F

考虑一个会变形的物体（假设为连续体），若受到外力（可能是表面力或是物体力），物体的内部就会有力的分布。物体内部的力会依循欧拉运动定律，正如物体受力依循牛顿运动定律一様。物体内部力的强度可以用应力来表示。因为物体假设为连续体，其内部的力也是会均匀分布在其体积中。

在工程中（例如结构工程、机械工程或土力工程）会透过应力分析来分析一物体中应力的的分布，例如隧道中岩石的应力，飞机机翼的应力，或是建筑物中梁柱的应力等。计算应力分布也就表示要知道物体中每一点的应力。据奥古斯丁·路易·柯西的理论，（假设为连续体的）物体中任何一点的应力（图2），可以完全由二阶(2,0)型的张量中的九个应力元素 $\sigma _{ij}$完全决定，此二阶张量称为柯西应力张量, ${\boldsymbol {\sigma }}$:

${\boldsymbol {\sigma }}=\left[{\begin{matrix}\sigma _{11}&\sigma _{12}&\sigma _{13}\\\sigma _{21}&\sigma _{22}&\sigma _{23}\\\sigma _{31}&\sigma _{32}&\sigma _{33}\\\end{matrix}}\right]\equiv \left[{\begin{matrix}\sigma _{xx}&\sigma _{xy}&\sigma _{xz}\\\sigma _{yx}&\sigma _{yy}&\sigma _{yz}\\\sigma _{zx}&\sigma _{zy}&\sigma _{zz}\\\end{matrix}}\right]\equiv \left[{\begin{matrix}\sigma _{x}&\tau _{xy}&\tau _{xz}\\\tau _{yx}&\sigma _{y}&\tau _{yz}\\\tau _{zx}&\tau _{zy}&\sigma _{z}\\\end{matrix}}\right]$

图3：连续体中的一点在平面应力条件下的应力转换

若确定了一物体在特定坐标系统$(x,y)$下的应力分布，有可能需要知道特定一点$P$相对另一个有旋转的坐标系统$(x',y')$下的应力张量，也就是在需要关注的点，在特定角度下的的应力张量。而此坐标系统$(x',y')$和原有的坐标系统$(x,y)$之间有一个角度差（图3）。例如，一般会需要知道最大的正向应力以及最大的剪应力，也需要知道其对应的方向。因此，需要发展一种张量转换的方式，可以配合坐标系统的旋转得到新坐标系统的张量。依照张量的定义，柯西应力张量遵守张量转换定律。应力的莫尔圆是用图解方式来说明柯西应力张量转换定律的方式。

二维张量下的莫尔圆

图4：连续体中的一点在平面应力条件下的应力分量

在二维下，一点$P$相对于垂直方向的应力张量可以用三个应力向量完全表示。在垂直坐标系统$(x,y)$下，其应力分量为：法向应力$\sigma _{x}$及$\sigma _{y}$，以及剪应力$\tau _{xy}$。由于角动量守恒，柯西应力张量会有对称性，也就是$\tau _{xy}=\tau _{yx}$，因此柯西应力张量可以写成：

${\boldsymbol {\sigma }}=\left[{\begin{matrix}\sigma _{x}&\tau _{xy}&0\\\tau _{xy}&\sigma _{y}&0\\0&0&0\\\end{matrix}}\right]\equiv \left[{\begin{matrix}\sigma _{x}&\tau _{xy}\\\tau _{xy}&\sigma _{y}\\\end{matrix}}\right]$

其目的是在另一个通过$P$点，但存在角度差的坐标系统$(x',y')$下，找到应力分量$\sigma _{\mathrm {n} }$及$\tau _{\mathrm {n} }$（图4）。坐标系统$(x',y')$和原坐标系统$(x,y)$的角度差即为$\theta$。

莫尔圆的方程

要推导二维平面应力及平面应变的莫尔圆方程，先考虑一个位在位置$P$的二维的无限小方形元素（图4），和$y$-$z$平面平行。

利用无限小元素上的力平衡，正向应力$\sigma _{\mathrm {n} }$及剪应力$\tau _{\mathrm {n} }$的大小为：

$\sigma _{\mathrm {n} }={\frac {1}{2}}(\sigma _{x}+\sigma _{y})+{\frac {1}{2}}(\sigma _{x}-\sigma _{y})\cos 2\theta +\tau _{xy}\sin 2\theta$

$\tau _{\mathrm {n} }=-{\frac {1}{2}}(\sigma _{x}-\sigma _{y})\sin 2\theta +\tau _{xy}\cos 2\theta$

莫尔圆参数式的推导－利用力平衡

利用$\sigma _{\mathrm {n} }$方向（$x'$轴）的力平衡（图4），而且假设$\sigma _{\mathrm {n} }$ 作用的面积为$dA$，可得： $\ {\begin{aligned}\sum F_{x'}&=\sigma _{\mathrm {n} }dA-\sigma _{x}dA\cos ^{2}\theta -\sigma _{y}dA\sin ^{2}\theta -\tau _{xy}dA\cos \theta \sin \theta -\tau _{xy}dA\sin \theta \cos \theta =0\\\sigma _{\mathrm {n} }&=\sigma _{x}\cos ^{2}\theta +\sigma _{y}\sin ^{2}\theta +2\tau _{xy}\sin \theta \cos \theta \\\end{aligned}}$ 再考虑以下的关系 $\cos ^{2}\theta ={\frac {1+\cos 2\theta }{2}},\qquad \sin ^{2}\theta ={\frac {1-\cos 2\theta }{2}}\qquad$ 及 $\qquad \sin 2\theta =2\sin \theta \cos \theta$ 可以得到 $\sigma _{\mathrm {n} }={\frac {1}{2}}(\sigma _{x}+\sigma _{y})+{\frac {1}{2}}(\sigma _{x}-\sigma _{y})\cos 2\theta +\tau _{xy}\sin 2\theta$ 再考虑$\tau _{\mathrm {n} }$方向（$y'$轴）的力平衡（图4），再假设 $\tau _{\mathrm {n} }$作用的面积是$dA$，可得： $\ {\begin{aligned}\sum F_{y'}&=\tau _{\mathrm {n} }dA+\sigma _{x}dA\cos \theta \sin \theta -\sigma _{y}dA\sin \theta \cos \theta -\tau _{xy}dA\cos ^{2}\theta +\tau _{xy}dA\sin ^{2}\theta =0\\\tau _{\mathrm {n} }&=-(\sigma _{x}-\sigma _{y})\sin \theta \cos \theta +\tau _{xy}\left(\cos ^{2}\theta -\sin ^{2}\theta \right)\\\end{aligned}}$ 再考虑以下的关系 $\cos ^{2}\theta -\sin ^{2}\theta =\cos 2\theta \qquad$ 及 $\qquad \sin 2\theta =2\sin \theta \cos \theta$ 可以得到 $\tau _{\mathrm {n} }=-{\frac {1}{2}}(\sigma _{x}-\sigma _{y})\sin 2\theta +\tau _{xy}\cos 2\theta$

上述二个方程也可以用柯西应力张量的张量变换定律来求得，这和在$\sigma _{\mathrm {n} }$及$\tau _{\mathrm {n} }$方向用力平衡计算是等效的。

莫尔圆参数式的推导－利用张量变换

应力张量变换定律可以表示为 ${\begin{aligned}{\boldsymbol {\sigma }}'&=\mathbf {A} {\boldsymbol {\sigma }}\mathbf {A} ^{T}\\\left[{\begin{matrix}\sigma _{x'}&\tau _{x'y'}\\\tau _{y'x'}&\sigma _{y'}\\\end{matrix}}\right]&=\left[{\begin{matrix}a_{x}&a_{xy}\\a_{yx}&a_{y}\\\end{matrix}}\right]\left[{\begin{matrix}\sigma _{x}&\tau _{xy}\\\tau _{yx}&\sigma _{y}\\\end{matrix}}\right]\left[{\begin{matrix}a_{x}&a_{yx}\\a_{xy}&a_{y}\\\end{matrix}}\right]\\&=\left[{\begin{matrix}\cos \theta &\sin \theta \\-\sin \theta &\cos \theta \\\end{matrix}}\right]\left[{\begin{matrix}\sigma _{x}&\tau _{xy}\\\tau _{yx}&\sigma _{y}\\\end{matrix}}\right]\left[{\begin{matrix}\cos \theta &-\sin \theta \\\sin \theta &\cos \theta \\\end{matrix}}\right]\end{aligned}}$ 将等号右侧展开，再配合$\sigma _{x'}=\sigma _{\mathrm {n} }$及$\tau _{x'y'}=\tau _{\mathrm {n} }$，可得： $\sigma _{\mathrm {n} }=\sigma _{x}\cos ^{2}\theta +\sigma _{y}\sin ^{2}\theta +2\tau _{xy}\sin \theta \cos \theta$ 再加上以下的条件 $\cos ^{2}\theta ={\frac {1+\cos 2\theta }{2}},\qquad \sin ^{2}\theta ={\frac {1-\cos 2\theta }{2}}\qquad$ 及$\qquad \sin 2\theta =2\sin \theta \cos \theta$ 可得 $\sigma _{\mathrm {n} }={\frac {1}{2}}(\sigma _{x}+\sigma _{y})+{\frac {1}{2}}(\sigma _{x}-\sigma _{y})\cos 2\theta +\tau _{xy}\sin 2\theta$ $\tau _{\mathrm {n} }=-(\sigma _{x}-\sigma _{y})\sin \theta \cos \theta +\tau _{xy}\left(\cos ^{2}\theta -\sin ^{2}\theta \right)$ 再加上以下的条件 $\cos ^{2}\theta -\sin ^{2}\theta =\cos 2\theta \qquad$ 及$\qquad \sin 2\theta =2\sin \theta \cos \theta$ 可得 $\tau _{\mathrm {n} }=-{\frac {1}{2}}(\sigma _{x}-\sigma _{y})\sin 2\theta +\tau _{xy}\cos 2\theta$ 此时不需要计算$\sigma _{x'}$垂直的应力成分$\sigma _{y'}$，因为在推导莫尔圆时还不需要此成分

这二个方程是莫尔圆的参数式。在方程中，$2\theta$为参数，而$\sigma _{\mathrm {n} }$和$\tau _{\mathrm {n} }$为坐标，因此表示若选择适当的坐标系统，使$\sigma _{\mathrm {n} }$为横轴，$\tau _{\mathrm {n} }$纵轴，给定参数$\theta$，会给定在莫尔圆上的一点。

若从参数式中消去参数$2\theta$，可以得到非参数式的莫尔圆方程。可以用重组$\sigma _{\mathrm {n} }$及$\tau _{\mathrm {n} }$的方程来达到。先将第一式等号右侧的第一项移到等号左边，二式平方后相加，可得

${\begin{aligned}\left[\sigma _{\mathrm {n} }-{\tfrac {1}{2}}(\sigma _{x}+\sigma _{y})\right]^{2}+\tau _{\mathrm {n} }^{2}&=\left[{\tfrac {1}{2}}(\sigma _{x}-\sigma _{y})\right]^{2}+\tau _{xy}^{2}\\(\sigma _{\mathrm {n} }-\sigma _{\mathrm {avg} })^{2}+\tau _{\mathrm {n} }^{2}&=R^{2}\end{aligned}}$

其中

$R={\sqrt {\left[{\tfrac {1}{2}}(\sigma _{x}-\sigma _{y})\right]^{2}+\tau _{xy}^{2}}}\quad {\text{and}}\quad \sigma _{\mathrm {avg} }={\tfrac {1}{2}}(\sigma _{x}+\sigma _{y})$

这就是圆（莫尔圆）的方程

$(x-a)^{2}+(y-b)^{2}=r^{2}$

在$(\sigma _{\mathrm {n} },\tau _{\mathrm {n} })$坐标系统中，其半径$r=R$，圆心在坐标$(a,b)=(\sigma _{\mathrm {avg} },0)$处。

符号体系

在使用莫尔圆时，需考虑两组分别的符号体系，一个是针对实体空间下应力分量的符号体系，另一个是针对“莫尔圆空间”下应力分量的符号体系。此外，工程力学（结构工程及机械工程）文献用的体系和地质力学用的符号体系不同。没有所有系统都适用的标准符号体系，是否要使用特定的符号体系取决于计算及诠释特定问题的方便程度。

上述图4的莫尔圆推导都是使用工程力学的符号体系，以下也会继续使用工程力学的符号体系。

实体空间符号体系

为了描述柯西应力张量的方便（图3及图4），应力分量的第一个下标表示应力分量作用的面，第二个下标表示应力分量的方向。因此$\tau _{xy}$是作用在以$x$轴正向为其法向量的平面上，而方向是往$y$轴的正方向。

在实体空间符号体系，正的正向应力是由作用平面往外（张力），负的正向应力是由作用平面往内（压缩力）（图5）。

在实体空间符号体系中，正剪应力在法向量为正的材料元素平面上，其作用方向会往轴的正方向，同样的，正剪力在法向量为负的材料元素平面上，其作用方向会往轴的负方向。例如作用在正向平面的剪应力$\tau _{xy}$和$\tau _{yx}$为正，因为这二个剪应力的作用方向往$y$轴及$x$轴的正方向（图3）。而相对应的作用在负向平面的剪应力$\tau _{xy}$和$\tau _{yx}$，其作用方向往$y$轴及$x$轴的负方向，因此这二个剪应力也为正。

莫尔圆空间符号体系

图5 绘制莫尔圆时，工程力学符号体系下的应力。此条目会依照图中的符号体系 # 3

在莫尔圆空间符号体系中，应力的符号体系和实体空间符号体系中的相同：正的正向应力是由作用平面往外（张力），负的正向应力是由作用平面往内（压缩力）

不过剪应力的符号体系和实体空间符号体系中的不同。在莫尔圆空间符号体系中，正的剪应力会使材料往逆时针方向旋转，而负的剪应力会使材料往顺时针方向旋转。因此在莫尔圆空间中，剪应力分量$\tau _{xy}$为正，而$\tau _{yx}$为负。这和实体空间符号体系中$\tau _{xy}$和$\tau _{yx}$符号相同的情形不同。

在绘制莫尔圆时，有二个作法可以绘制在数学上正确的莫尔圆：

1. 将正的剪应力画在上方（图5，符号体系#1）
2. 将正的剪应力画在下方，也就是$\tau _{\mathrm {n} }$轴倒置（图5，符号体系#2）

将正的剪应力画在上方会让莫尔圆上的$2\theta$角为正值时，旋转方向是顺时针旋转，这和实体空间符号体系中的相反。因此有些作者会选择让正的剪应力画在下方，这会让莫尔圆上的$2\theta$角为正值时，旋转方向是逆时针旋转，类似实体空间符号体系的情形。

为了克服剪应力轴往下才是正向的问题，有另外一种“替代的”符号体系，其中正的剪应力假设为将材料将顺时针方向旋转，而负的剪应力假设为将材料将逆时针方向旋转（图5，符号体系#3）。在“替代”体系下，正的剪应力轴往上，而且在莫尔圆上$2\theta$为正值时，旋转方向为逆时针。此符号体系产生的莫尔圆和图5，符号体系#2中的相同，因为正的剪应力$\tau _{\mathrm {n} }$也是会逆时针旋转的剪应力，也画在下方。而负的剪应力$\tau _{\mathrm {n} }$也是会顺时针旋转的剪应力，也画在上方。

此条目在实体空间符号体系中，会依照工程力学的符号体系，而在莫尔圆空间中，会使用“替代的”符号体系（图5，符号体系#3）。

绘制莫尔圆

图6：在平面应力及平面应变的条件下绘制莫尔圆（二倍角的作法）
在应力分析后，可以找到材料中一点$P$上的应力分量$\sigma _{x}$、$\sigma _{y}$及$\tau _{xy}$。应力分量作用在二个互相垂直的$A$平面及$B$平面，两者都通过$P$点。莫尔圆上$A$点和 $B$点的坐标是在$A$平面及$B$平面上的应力分量。因此可以用莫尔圆找到应力分量$\sigma _{\mathrm {n} }$及$\tau _{\mathrm {n} }$，也就是在同一点上，但作用在其他平面$D$上的应力分量。${\overline {OB}}$线和${\overline {OD}}$线之间的夹角是通过$P$点的平面$B$和平面$D$的法向量的夹角

假设已知待研究物体上的点$P$的应力分量$\sigma _{x}$、$\sigma _{y}$及$\tau _{xy}$，如图4所示。以下方法可以绘制点$P$的莫尔圆，以表示其应力状态。

1. 绘制笛卡尔坐标系统$(\sigma _{\mathrm {n} },\tau _{\mathrm {n} })$，横轴为$\sigma _{\mathrm {n} }$，纵轴为$\tau _{\mathrm {n} }$。
2. 在$(\sigma _{\mathrm {n} },\tau _{\mathrm {n} })$空间中，画出二点$A(\sigma _{y},\tau _{xy})$及$B(\sigma _{x},-\tau _{xy})$，分别是作用在二垂直平面$A$平面和 $B$平面上的应力分量（图4及图6），需依照选择的符号体系。
3. 用线段${\overline {AB}}$连接$A$点和$B$点，此即为圆的直径。
4. 绘制莫尔圆，其圆心$O$是线段${\overline {AB}}$的中点，也就是此线和$\sigma _{\mathrm {n} }$轴的交点。

找主要正向应力

主要应力的大小是点$C$和点$E$（图6中圆和 $\sigma _{\mathrm {n} }$轴的交点）中的横坐标。最大正向应力$\sigma _{1}$的大小恒为这二个横坐标中最大的那一个，而$\sigma _{2}$最小正向应力的大小恒为这二个横坐标中最小的那一个。这二个点的纵坐标为0，对应在主要平面上的剪应力为零，主要应力的大小也可以表示为

$\sigma _{1}=\sigma _{\max }=\sigma _{\text{avg}}+R$

$\sigma _{2}=\sigma _{\min }=\sigma _{\text{avg}}-R$

其中平均正向应力$\sigma _{\text{avg}}$的大小是圆心$O$的横坐标，为

$\sigma _{\text{avg}}={\tfrac {1}{2}}(\sigma _{x}+\sigma _{y})$

其半径的长度$R$为

$R={\sqrt {\left[{\tfrac {1}{2}}(\sigma _{x}-\sigma _{y})\right]^{2}+\tau _{xy}^{2}}}$

找最大和最小剪应力

最大剪应力和最小剪应力对应圆上最大及最小的纵坐标。这二个点是圆和通过圆心$O$的垂直线的交点。因此，最大和最小剪应力的大小为圆的半径$R$

$\tau _{\max ,\min }=\pm R$

找任意平面的应力分量

如前面所述，在二维应力分析后，可以知道在材料某一点$P$上的应力分量$\sigma _{x}$、$\sigma _{y}$及 $\tau _{xy}$。这些应力分量作用在通过$P$点的二垂直平面 $A$ 及 $B$，如图5及图6所述。莫尔圆也可以计算在莫尔圆上$D$的应力分量$\sigma _{\mathrm {n} }$及$\tau _{\mathrm {n} }$，事实是作用在$D$平面上，此平面也通过$P$点，和$B$平面有夹角$\theta$，计算应力分量有二种方式：倍角法以及平面原点法（origin of planes）

倍角法

如图6所示，若平面$D$是平面$B$再逆时针旋转角度$\theta$后的平面，要找到在平面$D$上的应力分量$(\sigma _{\mathrm {n} },\tau _{\mathrm {n} })$，可以在莫尔圆上从已知应力点 $B(\sigma _{x},-\tau _{xy})$同样以逆时针旋转，但旋转角度 $2\theta$，旋转到点$D(\sigma _{\mathrm {n} },\tau _{\mathrm {n} })$，也就是让${\overline {OB}}$线和${\overline {OD}}$线之间的夹角是$2\theta$。

倍角法的作法源自于通过$P$点的二实际平面之间的夹角$\theta$（图4），是其对应应力点 $(\sigma _{\mathrm {n} },\tau _{\mathrm {n} })$在莫尔圆上和圆心连线形成夹角的一半。

倍角关系是因为莫尔圆的参数式是$2\theta$的函数。也可以从在材料点 $P$上的平面$A$和$B$夹角是90度，而在莫尔圆上其应力点夹角为180度看出（90度的两倍）。

极点法（或平面原点法）

图7：平面应力及应变的莫尔圆（极点法）。从极点画的任何直线都会和莫尔圆相交，交点表示在和直线相同角度平面上的应力状态

第二种方式和要找到莫尔圆上的一个点，称为极点（pole）或是平面原点（origin of planes）。从极点画的任何直线都会和莫尔圆相交，交点表示在和直线相同角度的平面上的应力状态。因此若知道任何特定平面上的应力分量$\sigma$及$\tau$，可以画一条线通过莫尔圆上的 $\sigma _{\mathrm {n} }$和$\tau _{\mathrm {n} }$，且和平面平行，找到莫尔圆上这些线的交点，即为极点。例如，假设有应力状态如圆7所示，其分量是$\sigma _{x},\!$, $\sigma _{y},\!$及$\tau _{xy},\!$。首先先从$B$点画一条线，平行$\sigma _{x}$的作用平面，或是从$A$点画一条线，平行$\sigma _{y}$的作用平面，任一条线都会和莫尔圆交会，交会的点即为极点。在找到极点后，若要找到和垂直有$\theta$夹角的平面上的应力，可以从极点画一条平行该平面的线（见图7）。可以根据直线和莫尔圆的交点找到平面上的正向应力以及剪应力。

找主要平面的方向

最大主要应力及最小主要应力所在的平面方向也称为主要平面（principal planes），可以用莫尔圆中 的∠BOC及∠BOE判断，然后将二个角度都取一半。因此${\overline {OB}}$和${\overline {OC}}$之间的夹角是角∠BOC，是$\theta _{p}$（主要平面和平面$B$夹角）角度的二倍。

而$\theta _{p1}$和$\theta _{p2}$也可以用以下的方程取得

$\tan 2\theta _{\mathrm {p} }={\frac {2\tau _{xy}}{\sigma _{x}-\sigma _{y}}}$

此方程的解会是二个角度，彼此相差$90^{\circ }$。可以直接用圆的几何求解此方程，或是用圆的参数式，并且让$\tau _{\mathrm {n} }$等于零（主要平面上的剪应力为0）。

一般三维应力下的莫尔圆

图10 三维应力下的莫尔图

若要绘制三维应力下的莫尔图，需要先量测其主应力的大小$\left(\sigma _{1},\sigma _{2},\sigma _{3}\right)$以及方向$\left(n_{1},n_{2},n_{3}\right)$。

考虑以主应力轴为坐标系统，而不是用$x_{1}$, $x_{2}$, $x_{3}$坐标系统，并且假设$\sigma _{1}>\sigma _{2}>\sigma _{3}$，则在一法向量为 $\mathbf {n}$的平面，其应力向量$\mathbf {T} ^{(\mathbf {n} )}$的应力分量及剪力分量会满足下式

${\begin{aligned}\left(T^{(n)}\right)^{2}&=\sigma _{ij}\sigma _{ik}n_{j}n_{k}\\\sigma _{\mathrm {n} }^{2}+\tau _{\mathrm {n} }^{2}&=\sigma _{1}^{2}n_{1}^{2}+\sigma _{2}^{2}n_{2}^{2}+\sigma _{3}^{2}n_{3}^{2}\end{aligned}}$

$\sigma _{\mathrm {n} }=\sigma _{1}n_{1}^{2}+\sigma _{2}n_{2}^{2}+\sigma _{3}n_{3}^{2}.$

由于$n_{i}n_{i}=n_{1}^{2}+n_{2}^{2}+n_{3}^{2}=1$，可以用高斯消去法求解$n_{1}^{2}$, $n_{2}^{2}$, $n_{3}^{2}$：

${\begin{aligned}n_{1}^{2}&={\frac {\tau _{\mathrm {n} }^{2}+(\sigma _{\mathrm {n} }-\sigma _{2})(\sigma _{\mathrm {n} }-\sigma _{3})}{(\sigma _{1}-\sigma _{2})(\sigma _{1}-\sigma _{3})}}\geq 0\\n_{2}^{2}&={\frac {\tau _{\mathrm {n} }^{2}+(\sigma _{\mathrm {n} }-\sigma _{3})(\sigma _{\mathrm {n} }-\sigma _{1})}{(\sigma _{2}-\sigma _{3})(\sigma _{2}-\sigma _{1})}}\geq 0\\n_{3}^{2}&={\frac {\tau _{\mathrm {n} }^{2}+(\sigma _{\mathrm {n} }-\sigma _{1})(\sigma _{\mathrm {n} }-\sigma _{2})}{(\sigma _{3}-\sigma _{1})(\sigma _{3}-\sigma _{2})}}\geq 0.\end{aligned}}$

因为$\sigma _{1}>\sigma _{2}>\sigma _{3}$及$(n_{i})^{2}$都不是负值，因此其分子满足

$\tau _{\mathrm {n} }^{2}+(\sigma _{\mathrm {n} }-\sigma _{2})(\sigma _{\mathrm {n} }-\sigma _{3})\geq 0$ 因为其分母$\sigma _{1}-\sigma _{2}>0$而且$\sigma _{1}-\sigma _{3}>0$

$\tau _{\mathrm {n} }^{2}+(\sigma _{\mathrm {n} }-\sigma _{3})(\sigma _{\mathrm {n} }-\sigma _{1})\leq 0$ 因为其分母$\sigma _{2}-\sigma _{3}>0$而且$\sigma _{2}-\sigma _{1}<0$

$\tau _{\mathrm {n} }^{2}+(\sigma _{\mathrm {n} }-\sigma _{1})(\sigma _{\mathrm {n} }-\sigma _{2})\geq 0$ 因为其分母$\sigma _{3}-\sigma _{1}<0$而且$\sigma _{3}-\sigma _{2}<0.$

方程式可以写成

${\begin{aligned}\tau _{\mathrm {n} }^{2}+\left[\sigma _{\mathrm {n} }-{\tfrac {1}{2}}(\sigma _{2}+\sigma _{3})\right]^{2}\geq \left({\tfrac {1}{2}}(\sigma _{2}-\sigma _{3})\right)^{2}\\\tau _{\mathrm {n} }^{2}+\left[\sigma _{\mathrm {n} }-{\tfrac {1}{2}}(\sigma _{1}+\sigma _{3})\right]^{2}\leq \left({\tfrac {1}{2}}(\sigma _{1}-\sigma _{3})\right)^{2}\\\tau _{\mathrm {n} }^{2}+\left[\sigma _{\mathrm {n} }-{\tfrac {1}{2}}(\sigma _{1}+\sigma _{2})\right]^{2}\geq \left({\tfrac {1}{2}}(\sigma _{1}-\sigma _{2})\right)^{2}\\\end{aligned}}$

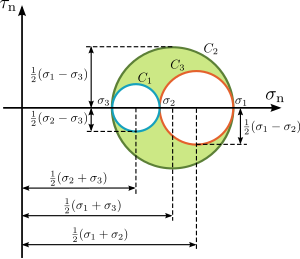
是三个应力莫尔圆$C_{1}$, $C_{2}$和$C_{3}$的方程，其半径分别是$R_{1}={\tfrac {1}{2}}(\sigma _{2}-\sigma _{3})$, $R_{2}={\tfrac {1}{2}}(\sigma _{1}-\sigma _{3})$及$R_{3}={\tfrac {1}{2}}(\sigma _{1}-\sigma _{2})$，而其圆心分别在$\left[{\tfrac {1}{2}}(\sigma _{2}+\sigma _{3}),0\right]$, $\left[{\tfrac {1}{2}}(\sigma _{1}+\sigma _{3}),0\right]$, $\left[{\tfrac {1}{2}}(\sigma _{1}+\sigma _{2}),0\right]$。

有了上述三个应力莫尔圆的方程，所有可能的应力点$(\sigma _{\mathrm {n} },\tau _{\mathrm {n} })$都会在三个应力莫尔圆之间的阴影区域（见图10）。应力点$(\sigma _{\mathrm {n} },\tau _{\mathrm {n} })$ 可能满足圆$C_{1}$的方程，或是在圆$C_{1}$的外面，可能满足圆$C_{2}$的方程，或是在圆$C_{2}$的里面，可能满足圆$C_{3}$的方程，或是在圆$C_{3}$的外面。

相关条目

• 临界面分析

参考资料

• Beer, Ferdinand Pierre; Elwood Russell Johnston; John T. DeWolf. Mechanics of Materials. McGraw-Hill Professional. 1992. ISBN 0-07-112939-1. 
• Brady, B.H.G.; E.T. Brown. Rock Mechanics For Underground Mining Third. Kluwer Academic Publisher. 1993: 17–29 [2018-02-05]. ISBN 0-412-47550-2. （原始内容存档于2020-08-07）. 
• Davis, R. O.; Selvadurai. A. P. S. Elasticity and geomechanics. Cambridge University Press. 1996: 16–26 [2018-02-05]. ISBN 0-521-49827-9. （原始内容存档于2020-08-07）. 
• Holtz, Robert D.; Kovacs, William D. An introduction to geotechnical engineering. Prentice-Hall civil engineering and engineering mechanics series. Prentice-Hall. 1981 [2018-02-05]. ISBN 0-13-484394-0. （原始内容存档于2019-06-08）. 
• Jaeger, John Conrad; Cook, N.G.W; Zimmerman, R.W. Fundamentals of rock mechanics Fourth. Wiley-Blackwell. 2007: 9–41 [2018-02-05]. ISBN 0-632-05759-9. （原始内容存档于2019-06-02）. 
• Jumikis, Alfreds R. Theoretical soil mechanics: with practical applications to soil mechanics and foundation engineering. Van Nostrand Reinhold Co. 1969 [2018-02-05]. ISBN 0-442-04199-3. （原始内容存档于2019-06-08）. 
• Parry, Richard Hawley Grey. Mohr circles, stress paths and geotechnics 2. Taylor & Francis. 2004: 1–30 [2018-02-05]. ISBN 0-415-27297-1. （原始内容存档于2020-08-07）. 
• Timoshenko, Stephen P.; James Norman Goodier. Theory of Elasticity Third. McGraw-Hill International Editions. 1970. ISBN 0-07-085805-5. 
• Timoshenko, Stephen P. History of strength of materials: with a brief account of the history of theory of elasticity and theory of structures. Dover Books on Physics. Dover Publications. 1983. ISBN 0-486-61187-6. 

外部链接

• Mohr's Circle and more circles by Rebecca Brannon （页面存档备份，存于互联网档案馆）
• DoITPoMS Teaching and Learning Package- "Stress Analysis and Mohr's Circle" （页面存档备份，存于互联网档案馆）