七角反棱柱
（重定向自正七角反棱柱）
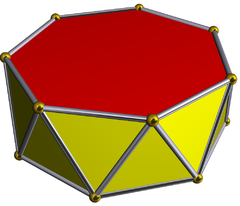
在几何学中，七角反棱柱又称为反七角柱或七角反柱是指底为七边形的反棱柱，侧面由三角形组成，若每一个面皆为正多边形则称为正七角反棱柱。每个七角反棱柱皆含有16个面[1][2][3]，是一种十六面体。
 | |||
| 类别 | 反棱柱 柱状均匀多面体 | ||
|---|---|---|---|
| 对偶多面体 | 七方偏方面体 | ||
| 识别 | |||
| 名称 | 正七角反棱柱 | ||
| 参考索引 | U77(e) | ||
| 鲍尔斯缩写 | heap | ||
| 数学表示法 | |||
| 考克斯特符号 |      | ||
| 施莱夫利符号 | sr{2,7} | ||
| 威佐夫符号 | | 2 2 7 | ||
| 康威表示法 | A7 | ||
| 性质 | |||
| 面 | 16 | ||
| 边 | 28 | ||
| 顶点 | 14 | ||
| 欧拉特征数 | F=16, E=28, V=14 （χ=2） | ||
| 组成与布局 | |||
| 面的种类 | 14个三角形 2个正七边形 | ||
| 面的布局 | 14{3}+2{7} | ||
| 顶点图 | 3.3.3.7 | ||
| 对称性 | |||
| 对称群 | D7d, [2+,14], (2*7), order 28 | ||
| 旋转对称群 | D7, [7,2]+, (722), order 14 | ||
| 特性 | |||
| 凸 | |||
| 图像 | |||
| |||
正七角反棱柱是基底为正七边形的七角反棱柱，其可视为一种半正多面体，施莱夫利符号s{2,7}表示其可以借由七边形二面体透过扭棱变换构造。其具有D7对称群[4]，其在威佐夫符号中用| 2 2 7表示[5]。
正七角反棱柱
编辑-
![正七角反棱柱[6]](//upload.wikimedia.org/wikipedia/commons/thumb/2/2e/Antiprism_7.png/120px-Antiprism_7.png)
正七角反棱柱[6]
当底面为正七边形时，会具备一些特别的性质
当基底边长为a的时候：
高：
表面积：
体积：
相关多面体与镶嵌
编辑| 对称群：[7,2], (*722) | [7,2]+, (722) | ||||||||
|---|---|---|---|---|---|---|---|---|---|
 
|
|
|
|
|
|
|
| ||
|
|
|
|
|
|
| |||
| {7,2} | t{7,2} | r{7,2} | 2t{7,2}=t{2,7} | 2r{7,2}={2,7} | rr{7,2} | tr{7,2} | sr{7,2} | ||
| 半正对偶 | |||||||||
|
|
|
|
|
|
|
| ||
|
|
|
|
|
|
|
|
| ||
| V72 | V142 | V72 | V4.4.7 | V27 | V4.4.7 | V4.4.14 | V3.3.3.7 | ||
| 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | n |
|---|---|---|---|---|---|---|---|---|---|---|---|
| s{2,4} sr{2,2} |
s{2,6} sr{2,3} |
s{2,8} sr{2,4} |
s{2,10} sr{2,5} |
s{2,12} sr{2,6} |
s{2,14} sr{2,7} |
s{2,16} sr{2,8} |
s{2,18} sr{2,9} |
s{2,20} sr{2,10} |
s{2,22} sr{2,11} |
s{2,24} sr{2,12} |
s{2,2n} sr{2,n} |
|
 
|
|
  
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
| |
| 作为球面镶嵌 | |||||||||||
|
|
|
|
|
|
|
|||||
在其他领域中
编辑参见
编辑参考文献
编辑- ^ Pugh, Anthony, Polyhedra: A Visual Approach, University of California Press: 21, 27, 62, 1976 [2014-06-22], ISBN 9780520030565, （原始内容存档于2014-07-09）.
- ^ heptagonal antiprism vertices （页面存档备份，存于互联网档案馆） wolframalpha.com [2014-06-22]
- ^ net of heptagonal antiprism （页面存档备份，存于互联网档案馆） korthalsaltes.com [2014-06-22]
- ^ Melnyk, Theodor William, Osvald Knop, and William Robert Smith. "Extremal arrangements of points and unit charges on a sphere: equilibrium configurations revisited." Canadian Journal of Chemistry 55.10 (1977): 1745-1761.
- ^ Heptagonal prisms and antiprisms （页面存档备份，存于互联网档案馆） umanitoba.ca [2014-6-22]
- ^ {7}-antiprism （页面存档备份，存于互联网档案馆） antiprism.com [2014-6-22]
- ^ Heptagonal Antiprism （页面存档备份，存于互联网档案馆） dmccooey.com [2014-6-22]
- Fowler, P. W., T. Tarnai, and Zs Gáspár. "From circle packing to covering on a sphere with antipodal constraints." Proceedings of the Royal Society of London. Series A: Mathematical, Physical and Engineering Sciences 458.2025 (2002): 2275-2287.
- heptagonal antiprism（页面存档备份，存于互联网档案馆） rediff.com [2014-6-22]